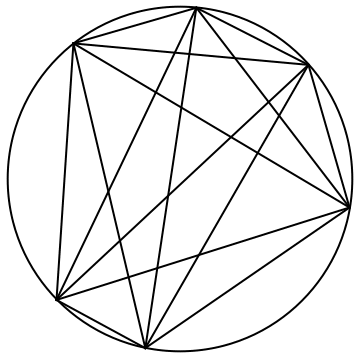
Problem of the Week 1099

Triangles Inside a Circle

Suppose that n ≥ 6 points are chosen on a circle, and subsequently each pair of points is connected by a line segment. Assuming that no three of these line segments meet at a single point, find the number of triangles formed, all of whose vertices lie entirely inside the circle. (In other words, we cannot use any of the original n points for our triangle.)

For example, the picture below shows n = 6, and exactly one triangle whose three vertices lie entirely within the circle.

Source: Charles Trigg, Mathematical Quickies, Dover, 1985.

© Copyright 2008 Andrew Beveridge and Stan Wagon. Reproduced with permission.