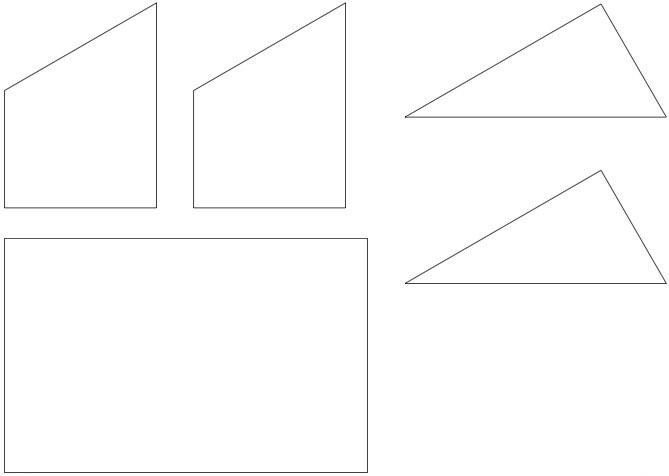
Problem of the Week 1186Coffin's Cruiser PuzzleLet R be a 48 × 31 rectangle. Let T be a 30°-60°-90° triangle with hypotenuse 34.565 (so legs are 17.2825 and 29.934). Let P be a right trapezoid with base 20.0982 and altitudes 27.1178 and 15.514:
Or, without the labels:
Show how to place two copies of T and two copies of P inside R with no overlap. Of course, you should not work with my numbers (which are useful for building a wooden model), but should cut out paper or, better, cardboard pieces from the template cited and try to make them fit. The fit is close to exact, so you might want to make R very slightly larger to handle possible inaccuracies in cutting.Source: Stewart Coffin, Cruiser puzzle, Geometric Puzzle Design, AKPeters, 2007. I had the pleasure of having dinner with Stewart here in Colorado a couple weeks ago. He showed me his wooden version of this puzzle, and of course that is the best material for a puzzle like this. One could also use a 3D printer. There is a danger that when making the box a little larger to accommodate construction inaccuracies one allows an unintended solution to arise. That in fact happened with Stewart's wooden model! I chose the numbers above to avoid such unintended solutions. © Copyright 2014 Stan Wagon. Reproduced with permission. |