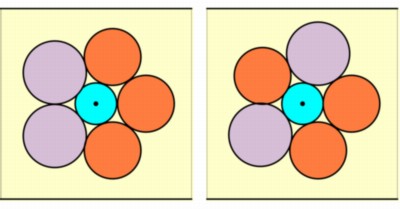
Problem of the Week 1137

A 13 Cent Problem

There are just two essentially different ways of arranging two nickels and three pennies in a ring so that each coin is tangent to two others and all five coins are externally tangent to a circle inside the ring.

For which arrangement is the radius of the inner circle larger?

While it is possible to answer the question knowing only that a nickel is larger than a penny, it is worthwhile to work out the radii in the two cases. The official radius of a U.S. penny is 9.525 mm; for a nickel it is 10.605 mm. Use these numbers to determine the radii of the two interior tangent circles that arise from the two types of rings, and the the difference in the two radii.

© Copyright 2010 Stan Wagon. Reproduced with permission.