Alice and Bob are to play a game on a six-button board, with the buttons arranged in a circle. One of the buttons is blue and the others are all red. Alice wins if the buttons ever become all red. The diagram shows the starting position.
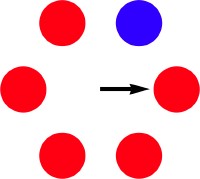
There is a clock hand that starts out pointing to the button next to (in clockwise direction) the blue one. The button the hand points to is called the active button. The clock hand rotates one step clockwise at each turn. The color of the active button determines whose turn it is: Blue means it is Alice's turn; red means Bob is to play. On a turn a player can choose to change the color of the active button, or to do nothing. After the choice is implemented, the clock hand advances one button clockwise.
Describe a strategy guaranteeing a win for Alice regardless of how Bob plays.
You will want to play the game live on a computer to get a feel for this: http://demonstrations.wolfram.com/ACombinatorialGame/. If you DOWNLOAD LIVE VERSION, you will get a file that will allow you to play. If you do not have Mathematica, you will need to download MathPlayer (free) from the same site in order to get the file to work for you.
© Copyright 2007 Stan Wagon. Reproduced with permission.